Is High-Order Wall-Modeled Large Eddy Simulation Ready for Prime Time?

During the past summer, AIAA successfully organized the 4th High Lift Prediction Workshop (HLPW-4) concurrently with the 3rd Geometry and Mesh Generation Workshop (GMGW-3), and the results are documented on a NASA website. For the first time in the workshop's history, scale-resolving approaches have been included in addition to the Reynolds Averaged Navier-Stokes (RANS) approach. Such approaches were covered by three Technology Focus Groups (TFGs): High Order Discretization, Hybrid RANS/LES, Wall-Modeled LES (WMLES) and Lattice-Boltzmann.

The benchmark problem is the well-known NASA high-lift Common Research Model (CRM-HL), which is shown in the following figure. It contains many difficult-to-mesh features such as narrow gaps and slat brackets. The Reynolds number based on the mean aerodynamic chord (MAC) is 5.49 million, which makes wall-resolved LES (WRLES) prohibitively expensive.

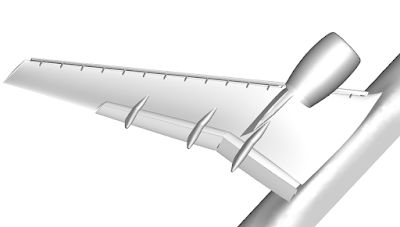The geometry of the high lift Common Research Model

University of Kansas (KU) participated in two TFGs: High Order Discretization and WMLES. We learned a lot during the productive discussions in both TFGs. Our workshop results demonstrated the potential of high-order LES in reducing the number of degrees of freedom (DOFs) but also contained some inconsistency in the surface oil-flow prediction. After the workshop, we continued to refine the WMLES methodology. With the addition of an explicit subgrid-scale (SGS) model, the wall-adapting local eddy-viscosity (WALE) model, and the use of an isotropic tetrahedral mesh produced by the Barcelona Supercomputing Center, we obtained very good results in comparison to the experimental data. 

At the angle of attack of 19.57 degrees (free-air), the computed surface oil flows agree well with the experiment with a 4th-order method using a mesh of 2 million isotropic tetrahedral elements (for a total of 42 million DOFs/equation), as shown in the following figures. The pizza-slice-like separations and the critical points on the engine nacelle are captured well. Almost all computations produced a separation bubble on top of the nacelle, which was not observed in the experiment. This difference may be caused by a wire near the tip of the nacelle used to trip the flow in the experiment. The computed lift coefficient is within 2.5% of the experimental value. A movie is shown here.        

Comparison of surface oil flows between computation and experiment 

Comparison of surface oil flows between computation and experiment 

Here are some lessons we learned from this case. Besides the space and time discretization methods, the computational mesh and the SGS model strongly affect WMLES results. 

• Since we obtain wall model data from the 2nd element away from the wall, it is important that isotropic elements be used near solid walls to ensure that turbulent eddies are resolved well there. That's why we prefer tetrahedral elements for complex geometries since one can always generate isotropic elements. In other words, inviscid meshes are preferred for WMLES!
  
  
• For very under-resolved turbulent flow, the use of an explicit SGS model such as WALE produces more accurate and robust results than a shock-capturing limiter. It is quite difficult to determine the appropriate amount of limiting.  

The recent progress has been documented in an AIAA Journal paper, and an upcoming conference paper in SciTech 2023. The latest high-order results indicate that high-order LES can reduce the total DOFs by an order of magnitude compared to 2nd order methods. We believe it is ready for prime time for high-lift configurations, turbomachinery, and race car aerodynamics. You are welcome to try high-order WMLES by getting the flow solver from www.hocfd.com.